What kind of geometric object is the Pauli spin matrix vector $\vec{\sigma} = (\sigma_1, \sigma_2, \sigma_3)$?

The Pauli spin vector $\vec\sigma$ relates a two-component spinor $s={\alpha\choose \beta}$ to its corresponding three-component vector $\vec{v}=(a,b,c)$. In Dirac bra-ket notation the relation is written as $$\vec{v}=\langle s|\vec\sigma|s\rangle,$$ in components $v_i=s^\ast\sigma_i s$. A pair of unitary operations $\pm U\in \text{SU}(2) $ on $s\mapsto s'$ is thereby mapped onto a rotation $R\in \text{SO}(3) $ of $\vec{v}\mapsto \vec{v}'=\langle s'|\vec\sigma|s'\rangle$. The mapping is illustrated by this table

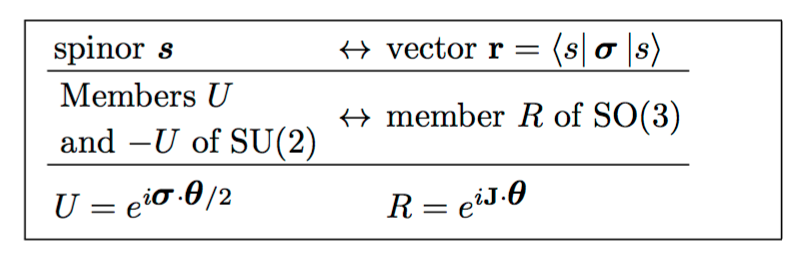
The usual vector identities do not apply to $\vec\sigma$, for example, instead of $\vec a\cdot(\vec b\times\vec a)=0$, one has $\vec \sigma\cdot(\vec v\times\vec \sigma)=-2i\,\vec v\cdot\vec\sigma$.

The Pauli spin vector encodes a two-complex-dimensional unitary representation of the Lie algebra $\bf{su}_2$. A basis of the Lie algebra maps to the matrix algebra $\bf{gl}_2(\mathbb{C}),$ giving a $3\times 2\times 2$ tensor. In 3 dimensions, physicists will sometimes blur the distinction between the basis for the tangent space and the Lie algebra of the Lorenz group $SU(2)$ at the origin, by the identification of $\hat{x}_i$ with the operator $\hat{x}_i\times -$ (this is not possible in higher dimensions), but these three matrices should really be thought of as parametrized by a basis for the Lie algebra.