Cardioid-looking curve, does it have a name?

The name of the curve is cochleoid (= shell-shaped rather than cardioid = heart-shaped).

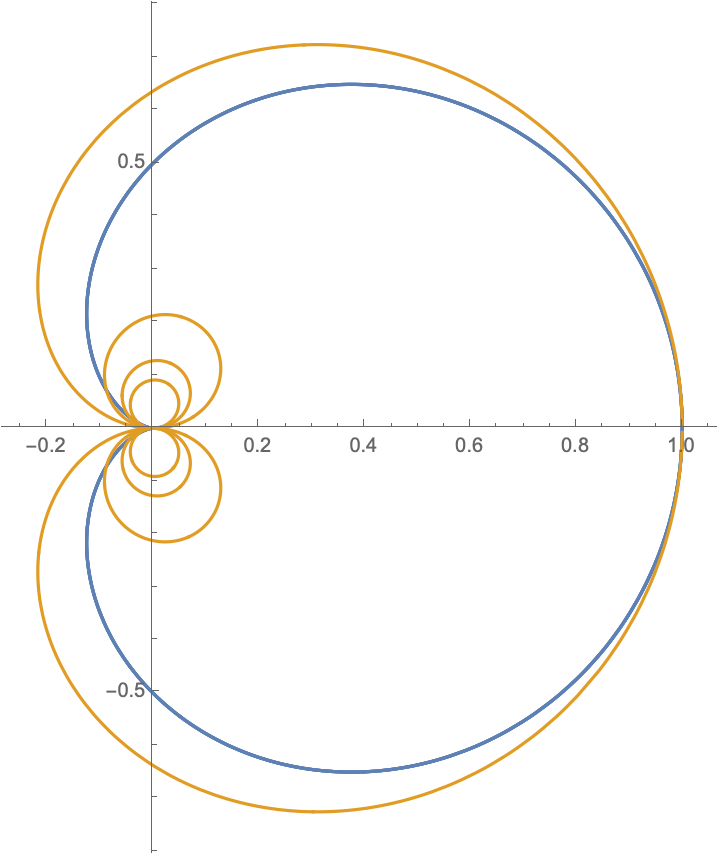
I compare the two below (gold = cochleoid, blue = cardioid). The distinction shell/heart refers to the additional windings remarked upon by მამუკა ჯიბლაძე , without these windings the two shapes would be qualitatively the same.

Not an answer - just want to note that the curve has more hidden branches. They can be seen looking at the parametric equation $$ x=\frac{\sin(\varphi)}\varphi,\quad y=\frac{1-\cos(\varphi)}\varphi $$ where $\varphi=2\theta$

From what LSpice and skbmoore shared https://mathcurve.com/courbes2d/cochleoid/cochleoid.shtml, There's this illustration of a helix on a cylinder The picture in the middle is the view when lined up with the side of the cylinder. If I understand the site correctly, the line traced by the helix in this perspective is a cochleoid.